A02. Minimal Information Content
There is not a whole lot to say about this chromatic scale.
– There are 12 steps (of theoretically, but not practically equal distance)
– After 12 steps the scale repeats, with every step doubling in frequency (this is called an octave)
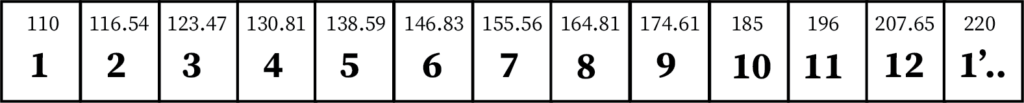
Alternatively, this could also just be expressed as a string of consecutive numbers:
1 2 3 4 5 6 7 8 9 10 11 12 (octave 1)
13 14 15 16 17 18 19 20 21 22 23 24 (octave 2)
25……etc
A little more practical visualization would be to imagine a piano keyboard – but one with white keys only. Let’s say we have about three octaves of chromatic scale – each octave repeats the same notes but at double the frequency. We usually perceive an octave as ‘the same note just higher’. On this imaginary (white keys only) keyboard we now have 36 (plus two extra) white keys with no markings. Unless we use the lowest (left-most) or highest (right-most) key and count keys there is no good way to find a specific note.

Imagine yourself the size of an ant, standing on one of these white keys somewhere in the middle. Looking forward, toward the higher pitches, or behind you, toward the lower pitches everything looks uniform, no matter on which white key you stand. This arrangement clearly doesn’t contain (or encode, or produce) a lot of information.